Prof. Dr. Otto Stern > Research Profile

by Luisa Bonolis
Otto Stern
Nobel Prize in Physics 1943
"for his contribution to the development of the molecular ray method and his discovery of the magnetic moment of the proton".
Becoming Einstein's Assistant
Otto Stern was born on February 17, 1888, in Upper Silesia. He studied physical chemistry at Breslau University, where he learned to master Boltzmann's work on statistical mechanics and Clausius' thermodynamics. In 1912, after earning his PhD, Stern went to work with Albert Einstein, who was then at Prague's German University. When Einstein, in October 1912, accepted a position at the ETH in Zurich, he invited Stern and appointed him his scientific assistant. At Prague and Zurich, Stern attended Einstein's lectures and learned from him what were the really important problems of contemporary physics.
At Zurich, Stern also met Max von Laue, who held a professorship there since 1912, the year of his discovery of X-ray diffraction from crystals, an achievement for which he would be awarded the 1914 Nobel Prize in Physics. During that period they became very good friends and when Niels Bohr's paper on the quantum model of the hydrogen atom appeared in 1913, they were both shocked by the novelty of the ideas propounded. Bohr had combined Ernest Rutherford's proposal of a dense positive nucleus orbited by negatively charged electrons with postulates about the quantization of the electron orbit and the emission and absorption of radiation. According to Bohr’s theory, electrons in an atom exist in stationary states: despite the fact that it is constantly accelerating, an electron moving in such allowed orbits, characterised by definite energy levels, does not radiate electromagnetic energy, thus its total energy En remains constant. Electrons can “jump” between different stationary states by losing or absorbing the appropriate energy, precisely equal to the difference of energy between quantum orbits. Electromagnetic radiation will be emitted of a frequency corresponding to such difference, divided by Planck's constant. Bohr's postulates not only explained the stability of atoms but also accounted for the by then well-established fact that atoms absorb and emit electromagnetic radiation only at specific frequencies.
This model, that enabled Bohr to calculate with remarkable accuracy the spectrum of atomic hydrogen, was such a departure from classical physics, that both von Laue and Stern vowed that, “If this nonsense of Bohr should prove to be right in the end, we will quit physics.” At the end of 1914, after Einstein left Zurich to become Director of the Kaiser Wilhelm Institut für Physik in Berlin, Stern followed von Laue to Frankfurt. After the war von Laue moved to Berlin University, and was substituted by Max Born, who "inherited'' Otto Stern as his assistant in the Institute for Theoretical Physics.
Molecular Beams
In 1911, the French physicist Louis Dunoyer had shown that atoms (sodium vapour, in this case) traveling in vacuum (at a pressure of about 10-3 millibar) produced on a wall cooled with liquid air a well-defined shadow image of an obstacle placed in their way. The image had exactly the shape calculated under the assumption that the molecules move in straight lines like rays of light, thereby confirming one of the key assumptions of the kinetic theory of gases. Such a beam was called a “molecular ray” or “molecular beam”. Stern was captivated by the “simplicity and directness” of the method, and immediately saw the vast possibilities of this discovery. In his Nobel lecture, he himself described the standard arrangement for producing molecular beams: “We have a vessel filled with gas or vapour, the oven. This vessel is closed except for a narrow slit, the oven slit. Through this slit the molecules escape into the surrounding larger vessel, which is continually evacuated so that the escaping molecules do not suffer any collisions. Now we have another narrow slit, the collimating slit, opposite and parallel to the oven slit. If the molecules really move in straight lines then the collimating slit should cut out a narrow beam whose cross section by simple geometry can be calculated from the dimensions of the slits and their distance.” Stern was fascinated by the possibility of measuring the properties of single atoms and molecules in gases and designed a first apparatus to produce direct evidence for the Maxwell-Boltzmann distribution of the velocity of gaseous molecules and to measure the mean velocity. Vacuum techniques in 1919 were still primitive compared with present ones, and the simple generation of a molecular beam was a major achievement. The kinetic theory gives quite definite numerical values for the velocity of the molecules depending on the temperature and the molecular weight. Stern's first experiment with molecular beams, completed in 1920, demonstrated in a simple, direct manner the fundamentals of the kinetic theory and that it was possible to prepare isolated molecules in a well-defined momentum state and thus to accurately measure any changes imparted to the molecules by external fields or other molecules. In laying the groundwork for much of what would come later, it represented an important step on the path to quantum physics.
The Stern-Gerlach Experiment: a Direct Confirmation of Spatial Quantisation
The first direct confirmation of Bohr's interpretation of spectral terms as stationary energy levels and of his frequency conditions was afforded by a series of experiments performed in Berlin by James Franck and Gustav Hertz in 1914. Electrons were accelerated to a known energy and directed against atoms of a gas or vapour at low pressure. At low electronic energies only elastic collisions occurred and no radiation was observed. As soon, however, as the electron's energy was equal to or exceeded a critical value (4.9 eV in the case of mercury vapour), inelastic collisions took place and radiation of a specific wavelength was observed. The energy loss of the electron corresponded to the energy difference between the ground state and the excited state of the atom, which, by transition to the ground state, re-emits this energy in the form of light in accordance with Bohr's frequency condition. Though Franck and Hertz claimed for some time that the 4.9 eV represented the ionization potential of mercury, Bohr considered the experiment to give strong support to his atomic theory, and argued rather: “It seems that their experiment may possibly be consistent with the assumption that this voltage corresponds only to the transition from the normal state to some stationary state of the neutral atom.” A decade later Franck and Hertz were awarded the 1925 Nobel Prize in Physics “For their discovery of the laws governing the impact of an electron upon an atom."
When atoms are in the presence of a magnetic field, some sharp spectral lines related to transitions between two energy states of different principal quantum number, split into multiple closely spaced lines of slightly different frequency, related to new separate energy states. Pieter Zeeman's discovery of the splitting of spectral lines in magnetic fields and Hendrik Lorentz's subsequent explanation, for which they shared the Nobel Prize in Physics 1902 “in recognition of the extraordinary service they rendered by their researches into the influence of magnetism upon radiation phenomena,” provided a triumph for the assumption of electrons being bound in atoms. In order to account for some Zeeman splittings of spectral lines, in 1916 Arnold Sommerfeld and Peter Debye had independently predicted on theoretical grounds that an external magnetic field provides a direction in space with respect to which Bohr's quasi-planetary electron orbits in the atom can orient themselves. As a consequence, the magnetic moment of the atom μ = e/2meL associated with the atomic angular momentum L due to the orbital motion of the electron, can only take certain discrete orientations in space with respect to the field; that is, its direction is “spatially quantised”. On the other side, there was the classical Larmor theorem, which was based on the assumption that the magnetic dipole moment vectors of the atoms would be randomly oriented and the angle with the external magnetic field could thus assume a continuous distribution of values.
Problems and puzzling questions related to spatial quantisation were widely discussed at the time. One morning, Stern woke up early and was still lying in bed when the thought came to him that the difficulty could be tackled directly by the molecular beam method. In an inhomogeneous magnetic field, whose inhomogeneity ∂H/∂z is normal to the beam direction, if theta designates the angle between the magnetic moment vector μ and the field gradient direction z, the atom will experience a force
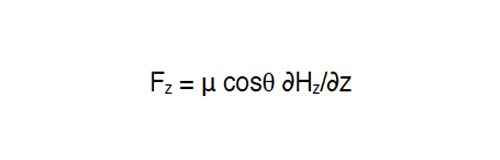
and will be deflected in the z direction by an amount that depends on the force, on the atomic mass and velocity, as well as on the length of the inhomogeneous field region. For the ground state, the space quantisation theory predicted that cos(theta) could assume only the values +1, 0, −1, and thus a splitting into discrete beams. According to classical mechanics, magnetic moments would instead remain randomly oriented, and thus a whole range of continuous values was to be expected for the z component of the magnetic moment, resulting in a continuous distribution of deflection angles, broadening - but not splitting - the beam.
Stern immediately discussed his idea with Born, who was not convinced that the space quantisation postulated by Sommerfeld and Debye, was something that really existed, taking it rather as “a kind of symbolic expression for something which you don't understand.” Debye himself did not think that spatial quantisation had a physical reality, merely being a “computational recipe,” for the additional magnetic energy of atoms in a magnetic field and accounting for spectroscopic evidence, while Sommerfeld oscillated between the random distribution and the three possible discrete positions. At the time Stern, too, did not believe in space quantisation. Both the classical and the quantum argument were strong. The experiment was really a question put to Nature, to use Stern's own words. It was a subtle but fundamental question indeed. In providing a proof of the existence of discrete energy levels, the Franck-Hertz result actually dealt with transitions between different short-lived states, connecting the electron quantum jumps with discrete energy differences, resulting in the emission of spectral lines. While Stern's project aimed at discovering whether different and permanent quantum states really existed. The experiment should answer this question unambiguously, demonstrating or refuting the existence of this new form of quantisation. Stern's abilities were rather in the direction of planning and evaluating experiments than in executing them, so he discussed his ideas with his Frankfurt colleague Walther Gerlach, an excellent experimentalist, who in 1912 had already tried to repeat Dunoyer's experiments without success.
As in Stern's first experiment, the molecular beam technique could provide direct and immediate information about molecules and atoms. The idea was that a collimated beam of silver atoms flowing from a tiny hole of a heated furnace and moving with thermal velocities should pass through a strong non-uniform magnetic field. The whole apparatus had to be so highly evacuated that practically no collisions would occur between the particles of the beam and those of the residual gas. Each beam particle being essentially isolated, beam slits, velocity selectors, magnetic fields, and/or oscillating fields, could be used to disturb them in a controlled fashion in order to determine their response to external disturbances.
The experiment was technically very challenging. The critical question was whether one could make a sufficiently inhomogeneous magnetic field in order to be able to observe the very small deflection of an atom carrying a magnetic moment of the order of a Bohr magneton, the natural quantum unit for expressing an electron magnetic dipole moment: μB = eh/2me (where e is the charge and me the mass of the electron). It was also necessary to define the beam to dimensions of about 0.1 mm so that with the available field gradient the deflection would be observable. Accordingly, the whole set up (oven, slits, magnet, condenser plate) had to be accurately aligned. The alignment had to be maintained for the several hours necessary to get a result from a molecular beam of such small intensity. A sufficiently low pressure had to be maintained so that the mean free path would be of the order of the beam length. Moreover, the core of the apparatus was subject to a large temperature gradient, as the beam source, a silver oven, was heated to about 1300 °C, while the mercury diffusion vacuum pumps and the condenser plate had to be cooled to the temperature of liquid air. Many difficulties had to be overcome, requiring a great amount of skill and perseverance.

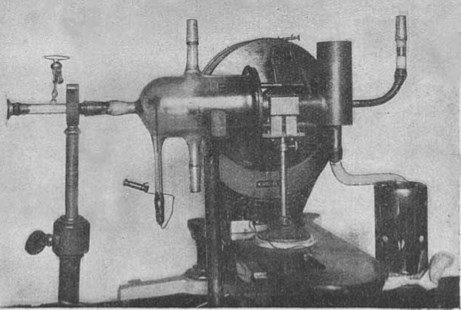
Max Born later recalled how he had tried to persuade Stern that there was no sense in performing such an experiment, but Stern replied that it was worth a try. One day, in early February 1921, the first proof of space quantisation appeared on the condenser plate. The parent beam split into two distinct parts, each corresponding to one distinct orientation for the silver atoms in the magnetic field, with no silver atoms in the central region. It appeared that in 1918 Bohr had been right. He had predicted a doublet splitting, corresponding to an electron orbiting around the atom nucleus clock-wise or counter-clockwise with respect to the direction of the magnetic field and resulting in only two discrete positions, parallel or antiparallel to the direction of the magnetic field. These two components would be respectively deflected upward and downwards, corresponding to cos(theta) = +1, − 1. Debye and Sommerfeld had predicted a triplet structure, in analogy to the normal Zeeman splitting, for the detected silver beam, with one component deflected downward, one upward, and one undeflected. Copies of a picture showing images of the silver beam deflection pattern without and with magnetic field were immediately sent to several physicists. Paschen's reply was: “This experiment proves for the first time the existence of Bohr's stationary quantum states.” A postcard was sent to Bohr: “We congratulate you on the confirmation of your theory.”
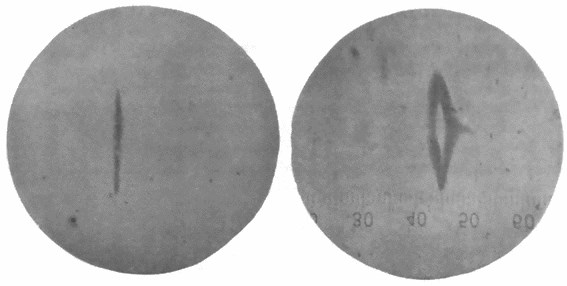
In providing “evidence that the silver atom possesses a magnetic moment,” Stern and Gerlach concluded that their results provided “direct experimental proof of the directional quantisation in a magnetic field.” Yet in spite of this apparently perfect agreement with theory, the Stern-Gerlach experiment led to grave difficulties related to the mechanisms of alignment of the atoms in the field, as pointed out by Einstein and Ehrenfest. No satisfactory answer could be given to the question: “How do the atoms take up their orientations?”
At the time, Stern and Gerlach considered their result a decisive refutation of the classical theory, which was based on continuous values for magnetic moments. But at the same time they mistakenly considered the phenomenon a confirmation of the old quantum theory, according to which the magnetic moment of the silver atom was due to the electrons' orbital angular momentum. The full significance of space quantisation could be realised only after the audacious proposal put forward in 1925 by Ralph de Laer Kronig, and a few months later by George Uhlenbeck and Samuel Goudsmit that the electron possesses an “internal angular momentum”, an intrinsic spin, and thus a related magnetic moment. Kronig was unfortunately dissuaded by Wolfgang Pauli from pursuing the idea of electron spin. In the silver atom there are 47 electrons surrounding the nucleus, of which 46 are arranged in a symmetrical cloud contributing nothing to the orbital angular momentum of the atom, and at the same time, the electrons with opposite spins cancel each other out, so that the total angular momentum (orbital + spin) is zero, and hence there is no magnetic moment due to the closed inner core. The forty-seventh unpaired electron also has zero orbital angular momentum, so the only source of magnetic moment is the intrinsic spin, a purely quantum mechanical effect, having no classical analogue. The beam of silver atoms divides in two, depending on the spin of the forty-seventh electron, so there are two possible states of spin which came to be known as up and down. A Stern-Gerlach apparatus can in fact be used to prepare a system in one state or another by filtering the output. One can for example block the beam of spin-down particles to produce a beam consisting of entirely spin-up particles.
However, at the time the Stern-Gerlach experiment was right in proving for the first time the reality of discrete, different and permanent quantum states, as postulated by Bohr. As a further remarkable result these experiments led to the first direct measurement of an atomic magnetic moment: the moment of the silver atom, which was found to be of the order of the Bohr magneton, a value predicted theoretically by Bohr for the moment of a hydrogen atom.
That same year Bohr would be awarded the 1922 Nobel Prize in Physics “for his services in the investigation of the structure of atoms and of the radiation emanating from them” and Einstein was awarded the 1921 Prize, not assigned the year before, for his quantum theory of the photoelectric effect.
Magnetic Moment of the Proton, Neutron and Deuteron
In 1922, the University of Hamburg offered Stern the position of full professor for Physical Chemistry. Until 1933, Stern's laboratory remained in the forefront of the art of making molecular beams and in a situation of virtual monopoly; furthermore, all successive molecular beam laboratories were founded by people who had learned from Stern, or by pupils of his pupils.
One of Stern's most ambitious scientific goals was the measurement of nuclear magnetic moments, which were estimated to be smaller than atomic ones by the ratio of the of the electron mass to the proton mass, that is “about 1/2000” of a Bohr magneton. At the beginning of the 1930s, measurements of nuclear spins and nuclear magnetic moments were supposed to provide important clues for the development of a consistent picture of the constitution of the atomic nucleus, a frontier area of physics, which attracted the interest of theoretical as well as experimental physicists. Up to that time, the only methods available for their determination were spectroscopic. Improvements in such techniques showed the existence of a further splitting of spectral lines into two or more components, the so-called hyperfine structure of spectra, whose most likely explanation was that it was due to interactions of the electronic shell with certain nuclear properties. However, these lines were very difficult to analyse in detail, being very close together and often not very well defined.
In spring 1932 Stern, who had aways been interested in fundamental questions, decided to try and measure the magnetic moment of the proton. This challenging investigation, which he carried out at an early stage with Otto Frisch, required tremendous improvements in the apparatus and an inhomogeneous magnetic field of sufficiently strong inhomogeneity and length to produce measurable effects. A new improved apparatus was built by Stern and Immanuel Estermann in November 1932.
Most people - including Stern - assumed that the result would simply found to be consistent with Dirac's relativistic quantum theory of the electron developed in 1928, that is a value about two thousand times smaller than the electron's magnetic moment. Pauli, in particular, who was in Hamburg as theoretician, thought that the experiment would be “a waste of time and effort, because the result is already perfectly known.” They found, to their surprise and to that of all other physicists, that it was more than twice as large as one would expect if the proton were a simple particle governed, as is the electron, by the Dirac wave equation. This experiment indicated that the proton is some kind of composite particle, a finding that was borne out by the important experiments on the structure of nucleons carried out by Robert Hofstadter during the 1950s, for which he would be awarded the Nobel Prize in Physics 1961.
The hydrogen atom has the most fundamental nucleus - the single proton - while deuterium has the simplest compound nucleus, consisting of a single proton and one neutron. After the success of the proton measurement, it was thus challenging to attempt to measure the magnetic moment of the deuterium nucleus. Since it was difficult to imagine that a neutral particle like the neutron should possess a magnetic moment, it was generally assumed that the deuteron would have the same magnetic moment as the proton. At this time, however, the political situation in Germany was deeply changing and in June 1933 Estermann was notified, together with other Jewish faculty members, that his tenure would be terminated as of 30 September 1933. Although Stern also was a Jew, his war service during the period 1915-1918 temporarily exempted him from the racial laws. Stern and Estermann worked in a great hurry, late into the night, but there was not enough time to get the detailed data they needed. They were only able to establish that the magnetic moment of the deuteron was only about one-third that of the proton. The only possible interpretation of these results was that the neutron must possess an intrinsic magnetic moment, which must be oriented in the opposite direction to that of the proton.
Stern's extremely successful work at Hamburg came to an end in August 1933 with his resignation. The pretext was the dismissal of his closest assistant Immanuel Estermann and an order to remove Einstein's portrait from his office. He emigrated with Estermann to the United States, where the Carnegie Institute of Technology at Pittsburgh, Pennsylvania, created for him a research professorship and he re-established there a molecular-beam laboratory. Their measurements on the nuclear magnetic moments initiated an important branch of nuclear physics and also opened an exciting series of experiments on the hydrogen isotopes later conducted by Stern and his collaborators and by Isidor Rabi at Columbia University in New York. After his stay in Hamburg in 1927, Rabi had become an enthusiastic follower of the molecular beam technique. He introduced new and powerful methods and founded a school that was able to achieve spectacular results in the coming years. The crowing achievement of Rabi's development of the molecular beam technique was the resonance method, from which a host of fundamental applications emerged and for which about 20 related Nobel Prizes were later awarded.
Only in the autumn of 1944, after the gap in the annual succession of Nobel Prizes due to the Second World War, the Swedish Academy of Sciences announced that for 1943 the Prize in Physics would be awarded to Otto Stern, “for his contribution to the development of the molecular ray method and his discovery of the magnetic moment of the proton.” There is however no mention of the Stern-Gerlach experiment. The 1944 Prize was awarded to Isidor Rabi, “for his resonance method for recording the magnetic properties of atomic nuclei.”
Bibliography
Bretislav, F. and Herschbach, D. (2003) Stern and Gerlach: How a Bad Cigar Helped Reorient Atomic Physics. Physics Today 56: 53-59
Estermann, I. and Foner, S. N. (1975) History of molecular beam research: Personal reminiscences of the important evolutionary period 1919–1933. American Journal of Physics 43: 661- 671
Rabi, I. I. (1969) Otto Stern, Co-discoverer of Space Quantization, Dies at 81. Physics Today 22: 103-104
Rigden, J. S. (1983) Molecular Beam Experiments on the Hydrogens during the 1930s. Historical Studies in the Physical Sciences 13(2): 335-373
Segrè, E. (1973) Otto Stern: 1888-1969. Biographical Memoir of the National Academy of Sciences: 213-236
Stern, O. Interview by Thomas S. Kuhn. May 29 and 30, 1962. American Institute of Physics, http://www.aip.org/history/ohilist/4904.html
Toennies, J.P. et al. (2011) Otto Stern (1888-1969): The founding father of experimental atomic physics. Annalen der Physik 523(12):1045-1070
Weinert, F (1995) Wrong Theory-Right Experiment: The Significance of the Stern-Gerlach-Experiments. Studies in History and Philosophy of Modern Physics 26: 75-86.
