Prof. Dr. Niels Henrik David Bohr > Research Profile

by Roberto Lalli

Niels Henrik David Bohr

Nobel Prize in Physics 1922
"for his services in the investigation of the structure of atoms and of the radiation emanating from them".

Born in Copenhagen on October 7 1885, Niels Bohr was the second of three children of the eminent Danish physiologist Christian Bohr. Since his boyhood, Bohr enjoyed the lively cultural environment surrounding his father’s professional life. Even before becoming a university student, Bohr attended gatherings with important exponents of the Danish intelligentsia, such as the philosopher Harald Høffding, who had a long-lasting influence on the philosophical outlook which Niels Bohr brought to physical problems. He began his studies in physics in 1903 at the University of Copenhagen, then the only university in Denmark. Although Bohr is remembered for his fundamental contributions to theoretical physics, his first work concerned the purely experimental question of measuring the surface tension of a jet of liquid emerging from a cylindrical tube. To deal with this question, Bohr had to deepen both the experimental and theoretical issues concerning such a calculation. The mixture of theoretical ability and physical intuition that Bohr showed in handling this problem remained one the central features of Bohr’s style.

Bohr began his epoch-making study of the structure of matter with his master’s thesis on the electron theory of metals - a topic that he further elaborated in his PhD dissertation completed in 1911. The theory on which Bohr based his study was the Lorentz-Drude model, according to which metals were depicted as gases of electrons moving almost freely in a potential generated by positive charged ions fixed in a crystal structure. The Lorentz-Drude theory explained some of the electrical and thermal properties of metals, but several experiments disagreed with the values predicted by the theory. By generalizing the assumptions of the Lorentz-Drude theory, Bohr deduced that it was not possible to derive the diamagnetic and paramagnetic properties of metals from the accepted laws of electromagnetism. This conclusion was fundamental in giving Bohr the conviction that a revision of classic electromagnetism was necessary, in order to deal with atomic phenomena. The problem Bohr underlined in his dissertation was, indeed, resolved only after fundamental developments of quantum theory, such as the formulation of the exclusion principle by Wolfgang Pauli in 1925 and the independent development by Enrico Fermi and Paul Dirac of the statistics of the particles obeying said principle in 1926.

Inside the Atom

The following steps of Bohr’s intellectual life concerned his research in England with two of the most authoritative experimental physicists of the period: J. J. Thomson, who had received the Nobel Prize in Physics in 1906 for his discovery of the electron; and E. Rutherford, who had been awarded the Nobel Prize in Chemistry in 1908 for his studies on radioactivity. Both Thomson and Rutherford had established two flourishing schools of experimental physics housed in two different laboratories. The former succeeded Lord Rayleigh as the third director of the Cavendish Laboratory in Cambridge in 1884, while the latter had instituted his laboratory in Manchester in 1907. They had also formulated two different models of the atom. Thomson had been building the first well-known dynamical model of the atom since 1903. At that time, electrons were the only subatomic particles whose existence was widely accepted because of various experimental observations, culminating in Thomson’s verification of the constancy of the electron charge-mass ratio in 1897. In the Thomson model, the negatively charged electrons were the only corpuscular constituents of the atom, while the electrical neutrality was obtained by hypothesizing a substance that surrounded the electrons and whose positive charge perfectly balanced that of the atomic electrons.

Rutherford proposed a different model in 1911 after the result of the Geiger-Marsden experiments performed at the Manchester laboratory had convinced him that all the positive charge was concentrated in the pointlike centre of the atom, which he later called the nucleus. Rutherford hypothesized a planetary model of the atom in which a sphere of negative electrification of charge –Ne (where e is the charge of the electron) surrounded the nucleus of total charge +Ne due to the attraction generated by the Coulomb potential of the nucleus. In his proposal of the nuclear atom, however, Rutherford did not attempt to resolve the theoretical issues concerning the mechanical and electromagnetic stability of the atom. The major outcomes of Rutherford’s proposal were the clarification of the role of the nucleus in the scattering of alpha particles as well as of its contribution to the total atomic mass. In spite of its success in explaining some specific experimental results, the Rutherford atom lacked the mathematical refinements of the Thomson model and was rarely cited by the scientific community in the period 1911-1913.

The main developments of the Rutherford model were due to Bohr who used the study of the structure of the atom to pursue that departure from classical electromagnetism he had envisaged in his dissertation. This research was Bohr’s earliest relevant contribution to the development of quantum physics. Bohr started by underlining the problems affecting the dynamics of the electrons in Rutherford’s nuclear model. Such a system, Bohr maintained, was unstable both electromagnetically and mechanically. The former instability depended on the radiation loss due to the accelerated motion of the electrons, which would eventually lead the electron to fall on the nucleus. The mechanical instability occurred in atoms with more than one electron, because of the repulsive forces between the electrons moving in the same orbit. To overcome these difficulties, Bohr introduced a novel “hypothesis for which there will be no attempt at a mechanical foundation (as it seems hopeless)” - a hypothesis in which the kinetic energies of the electrons rotating around the nucleus were related to the period of rotation through a constant that, in turn, depended on Planck’s quantum of action. In 1913, Bohr published his proposal in three long papers grouped under the same name “On the Constitution of Atoms and Molecules.” The first of the three papers dealt with the constitution of the hydrogen atom and was a milestone in the development of the old quantum theory. The main hypothesis on which Bohr based his model was that the electron in its ground state is stable and is not subject to a radiative loss – an assumption that resolved the problem of the electromagnetic instability affecting the Rutherford model. Moreover, Bohr related this postulate to the observed spectral lines of the hydrogen atom synthesized by the generalized Balmer formula. Bohr postulated that the electron of the hydrogen atom could only occupy stationary states of specific energies. In order to explain the discreteness of atomic spectra, Bohr formulated a second postulate that constituted a deep break with classical electromagnetism: The hydrogen atom emits or absorbs radiation if and only if the electron goes from a stationary state to another by means of a sudden jump: the spectrum is discrete because the allowed stationary states are discrete. The frequency of the radiation emitted was a pure quantum phenomenon and was related to the difference of energies between the states 1 and 2 through the Planck constant h:

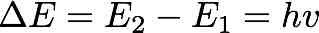
With this first formulation of a quantum model of the hydrogen atom, Bohr was able to resolve the theoretical problem of the stability of the atom, while explaining the discreteness of spectra. He also derived the Rydberg constant from the atomic constants and thus was able to explicitly write the generalized Balmer formula for the emission frequencies of the the hydrogen atom:

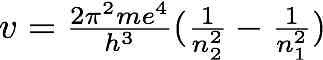
where m and e are respectively the mass and the charge of the electron.

Bohr’s formula (2) was extremely successful in explaining the observed spectra of the hydrogen-like atoms. Moreover, in 1914 Bohr’s theory of the atom received the most striking confirmation by means of an experiment performed by J. Franck and G. Hertz who observed the behavior of electrons passing through a low pressure gas in dependence of their energy. Franck and Hertz observed that up to a specific energy level the electrons were elastically scattered; however, as soon as the energy of the electrons exceeded this value the scattering became inelastic and a specific spectral line was observed. This observation was soon interpreted as a confirmation of the existence of energy levels within the atoms of the target gas.

The Correspondence Principle

The next fundamental contribute Bohr made to the quantum theory concerned the formulation of the correspondence principle, which was much more complex and controversial than the one usually exposed in physics textbooks. Bohr did not intend the principle, indeed, as a general agreement between the predictions of quantum and classical theories in the classical limit, but as specific formal correlations between quantum theory and classical mechanics. Already in 1913, Bohr used in the derivation of the Rydberg constant a reasoning that presented some analogies with what he later called the principle of correspondence. With his formula of the radiation emitted by atoms, Bohr had conceptually separated the mechanical frequency of the periodic motion of the electrons (whose harmonics, according to classical electrodynamics, should correspond to the frequencies emitted by the atom), from the radiated frequency that depended only on the energy difference between two stationary states (a pure quantum phenomenon). Bohr contended that, for high quantum numbers, the radiated frequency of the transition between two states n and n-τ was almost equal to the harmonic τ of the mechanical orbital frequency of the initial stationary state. In 1918, Bohr proposed the first systematic generalization of the correspondence principle in the first part of his paper “On the Quantum Theory of Line Spectra,” where he used this generalization to derive the selection rules in the Zeeman and Stark effects and in the fine structure of the hydrogen atom. Bohr extended the principle to multiperiodic systems (physical systems whose dynamics is determined by more than one fundamental frequency) and related the classical electrodynamical derivations of the polarization and intensities of the spectral lines to the probabilities of transition between specific stationary states of the quantum atom.

Although the agreement between quantum probabilities and classical Fourier coefficients was derived only for high quantum numbers, Bohr contended that the amplitude of the harmonic vibrations would “in some way give a measure for the probability of a transition between two states,” also for small quantum numbers. His collaborator Hans Kramers soon utilized the principle to successfully derive the approximate intensities and the polarizations of the lines of the hydrogen spectrum. Only in 1920, however, Bohr eventually exposed a definition of the correspondence principle: "Although the process of radiation cannot be described on the basis of the ordinary theory of electrodynamics, according to which the nature of the radiation emitted by an atom is directly related to the harmonic components occurring in the motion of the system, there is found, nevertheless, to exist a far-reaching correspondence between the various types of possible transitions between the stationary states on the one hand and the various harmonic components of the motion on the other hand. This correspondence is of such a nature, that the present theory of spectra is in a certain sense to be regarded as a rational generalization of the ordinary theory of radiation."

After Bohr had explicitly related the quantum theory to the “formal analogy” with classical mechanics and electromagnetism, the principle became a heuristic conceptual device that came to govern the development of the old quantum theory and provided the basis for Heisenberg’s first formulation of matrix mechanics in 1925. The significance of the principle was in turn related to the creation of the school of theoretical physics in Copenhagen directed by Bohr and institutionalized in 1921 with the establishment of the University Institute of Theoretical Physics. During the 1920s, the Institute became one of the major poles of attraction for young theoretical physicists, including Paul Dirac, Pascual Jordan, Werner Heisenberg, Wolfgang Pauli, and John Slater. The Institute generated a community of theoreticians that focused on the same problems and often used a similar approach. The success of this approach was internationally recognized, culminating in the Nobel Prize in physics awarded to Niels Bohr in 1922 "for his services in the investigation of the structure of atoms and of the radiation emanating from them." Bohr himself used the correspondence principle in 1921 to deal with polyelectronic atoms. Having more than one electron, such atoms were assumed not to be multiperiodic. However, the polyelectronic atoms showed experimental properties quite similar to the hydrogen atom. In particular, the atoms all had discrete spectra. This feature led Bohr to apply the correspondence principle in order to define the structure of all the atoms in 1922. In Bohr’s "second atomic theory," as the model was later called, Bohr introduced the idea that in the state of minimum energy, the electrons were in orbits of different quantum numbers - an idea that led Bohr to explain the periodicity of the table of elements. The success of this idea depended also on experimental verifications, including the discovery by Dirk Coster and George de Hevesy of the missing element 72 (Hafnium), following Bohr’s indication that its chemical properties should not resemble those of the rare earths but rather be similar to those of element 40 (Zirconium).

The Road to Quantum Mechanics

Bohr’s influence on the development of quantum theory was not limited to the researches awarded with the Nobel Prize. The correspondence principle continued to guide the researches of various theoretical physicists who were trying to overcome the difficulties of old quantum theory in the explanation of the Helium spectrum and of the anomalous Zeeman effect. By applying the correspondence principle to the derivation of the optical dispersion formula, Kramers introduced a formalism, which was later developed by Heisenberg in a general mathematical scheme in the first formulation of matrix mechanics. In 1924, Bohr himself contributed to the theoretical debate by putting forward a new theory of radiation along with Kramers and Slater, later called the BKS theory. Before the discovery of the Compton effect in 1923, Einstein’s proposal that the radiation itself was quantized was considered too bold because it conflicted with the well-known interference phenomena of the electromagnetic field, and Bohr himself had publicly rejected Einstein’s hypothesis. In 1924, Bohr began looking for a new theory of radiation processes that explained the Compton effect, while maintaining the essential dualism between the discrete nature of matter and the continuous nature of the radiation field. According to the BKS theory, a virtual radiation was directly emitted by the electron in a stationary state. In this new picture, to the harmonic component τ of the nth stationary state corresponded a virtual oscillator, which emitted or absorbed a virtual field governed by the Maxwell equation in free space. The virtuality of oscillations and fields depended on the fact that they were accessible only statistically by observing transitions in a large number of atoms. The deepest feature of the BKS theory was that atoms influence each other only probabilistically. This meant that the classical principle of the energy-momentum conservation was violated. In the BKS theory the total energy-momentum was conserved only statistically, not for individual processes. Related to this failure of the energy-momentum conservation for individual processes was the renunciation of a causal connection between transitions in distant atoms. The BKS theory was eventually proved wrong by the experimental confirmation of the exact conservation of energy-momentum in the scattering between radiation and individual electrons. However, some commentators maintain that the theory had a role in the development of quantum mechanics because it provided a physical picture of the dispersion theory of Kramers and Heisenberg. More conceptually, the BKS theory paved the way to the following developments of the concept of probability in quantum mechanics. In an interview released in 1963, Heisenberg referred to the BKS theory as a “central step,” in the development of quantum mechanics because in such a theory the virtual waves “were a physical reality in the sense that they produced probabilities for decay or emission, and, at the same time [...] were not completely real like the electromagnetic waves.“ For Heisenberg, “such intermediate kind of reality was just the price which one had to pay for understanding quantum theory.”

The last great fundamental contribution of Niels Bohr to quantum mechanics was the enunciation of the principle of complementarity that, along with Heisenberg’s principle of indeterminacy, constituted the core of the Copenhagen interpretation of quantum mechanics, which remained the main interpretative approach to quantum mechanics for several decades. The two principles followed a development of the theory in which the competing pictures of Heisenberg’s matrix mechanics and Schrodinger’s wave mechanics had been proved to be formally identical, the statistical interpretation of the wave function proposed by Max Born in 1925 had gained momentum within the community of theorists, and the Dirac-Jordan transformation theory had provided the necessary formal generalization that unified the diverse approaches in a coherent conceptual apparatus. The new formalism, however, lacked a clear physical picture for all the mathematical symbols employed in the calculations. As Heisenberg recognized, the conceptual problems surrounding quantum mechanics in 1926 stemmed from the impossibility of applying ordinary conceptual schemes to describe atomic processes. In particular, the space-time descriptions as well as the possibility to conceive casual connections of physical phenomena were prevented by some specific features of the quantum-mechanical formalism, such as the employment of non-commutative quantities and the use of abstract multi-dimensional spaces.

While Heisenberg took the decisive step to define the mathematical relationship that limited the precision with which the values of position and linear momentum could be simultaneously known, Bohr tried to formulate a coherent physical view of quantum mechanics through the principle of complementarity. Bohr was trying to resolve the conceptual problems concerning the particle-wave duality of light. According to many, this contradiction was inextricably associated with the De Broglie formulas for the corpuscular energy and momentum, which were based on the wave concepts of frequency and wave number. This contradiction had led Bohr to discard Einstein’s proposal of the photon and to propose the alternative BKS theory. After the Compton effect was confirmed for individual processes, Bohr began looking for a resolution of the contradiction inherent in wave-particle duality. Starting from these considerations, Bohr matured the idea that both features of the phenomena were essential, although mutually exclusive. In this view, the uncertainty relation calculated by Heisenberg became the measure of the impossibility that a physical situation could show simultaneously the two complementary aspects of the phenomena.

In his first exposition of the principle, Bohr stated “[t]he very nature of quantum theory […] forces us to regard the space-time coordination and the claim of causality, the union of which characterizes the classical theories, as complementary but exclusive features of the description, symbolizing the idealization of observation and definition respectively.” In Bohr’s views, the apparent contradiction of the wave-particle duality stemmed from the “impossibility of any sharp separation between the behavior of atomic objects and the interaction with the measuring instruments which serve to define the conditions under which the phenomena appear.” Several physicists regarded the complementary principle as an interpretation of quantum phenomena that allowed the consistent application of classical concepts and that clarified the relationships between these concepts and the experimental conditions, which entered within the formal structure of the quantum theory. With time, the Copenhagen interpretation became the orthodox interpretation of quantum mechanics and influenced several generations of physics. However, it is not possible to briefly summarize the conceptual meaning of the principle: Bohr himself continued to struggle for a coherent and complete definition of the principle and its interpretation has led to several philosophical and physical controversies.

Inside the Nucleus

The last major contributions of Niels Bohr to physics appeared in the 1930s in the field of nuclear physics. In 1913, Bohr had already touched on this topic by arguing that, according to his atomic theory, the β-decay had necessarily to be a nuclear phenomenon. He had reached this conclusion through a comparison between the chemical properties of the isotopes and the spectrum of their β-decay. While isotopes of the same elements had identical chemical properties, and, consequently, the same electronic configurations, they emitted β electrons with different velocities. Consequently, Bohr concluded, these emissions had nothing to do with the orbital electrons.

From the 1910s to the early 1930s, the models of the nucleus were based on the idea that its constituents were only the protons and the electrons. The discovery of the neutron by the English physicist James Chadwick in 1932, allowed a reconfiguration of the theoretical models that simplified the theoretical explanation of some experimental features of the atoms, such as the relationship between the atomic mass and the atomic number of the elements, the statistics followed by the nuclei, and their observed spin.

The discovery of the neutron did not have momentous consequences only from a theoretical perspective, but it also produced deep transformations in the experimental practice. From 1932 onward, it was possible to use neutrons in order to penetrate the nuclei, because neutrons carry no charge and, consequently, are much more penetrating than, for example, alpha particles. Bohr was the first theoretical physicist to propose a reliable model that aimed at explaining the scattering observed after the nuclei had been bombarded by neutrons. In 1935, Bohr proposed that nuclear reactions should be interpreted as a two-stage process. In the first stage, the projectile amalgamates with the nucleus and forms what Bohr later called the “compound nucleus,” subject to quantum mechanical fluctuations. The second stage occurs after a certain lapse of time and could lead to three different outcomes: 1) the compound nucleus disintegrates into the particles which originally formed it, with the nucleus maintaining its initial energetic configuration; 2) the compound nucleus breaks up into the original particles, but the original nucleus has gone to one of its excited energy status; 3) the compound nucleus separates into particles different from the initial ones.

Along with the Danish physicist Fritz Kalckar, Bohr developed his nuclear model and proposed a parallel between the nucleus and a drop of liquid - a parallel already proposed in 1928 by George Gamow. Bohr’s liquid drop model considered the nucleus as a collection of nucleons in which the repulsive electromagnetic forces between protons were counter-balanced by strong short-range attractive forces. The nucleus, when excited by, for example, an incoming neutron, produces different kind of vibrations, similar to the surface and volume vibrations of a drop of liquid. For small energies of the incoming neutrons, Bohr and Kalckar calculated that nuclear excitations corresponded to quantized surface vibrations of the compound nucleus described by the nuclear spectrum. This model dominated the theoretical researches on the behavior of the nucleus up to the early 1950s, when it was substituted by the shell nuclear model developed independently by several physicists, including Eugene Wigner, Maria Goeppert-Mayer, and J. Hans D. Jensen, who shared the Nobel Prize in Physics in 1963.

In 1938, Otto R. Frish and Lise Meitner employed the liquid drop model to explain the surprising discovery made by Otto Hahn and Fritz Strassmann that uranium seemed to have split into two lighter elements after neutron bombardment. Meitner and Frisch's analysis marked the beginning of the theoretical studies on nuclear fission. Bohr soon agreed with their approach and improved the application of the liquid-drop model by producing, along with John A. Wheeler, the first detailed quantitative study of the mechanism of the fission processes in 1939. One of the main successes of their theoretical model was the prediction that the rare isotope of Uranium U-235 was fissile by slow neutrons, while the more common isotope U-238 tended to absorb the incoming neutron for small velocities.

Bohr’s papers on nuclear fission were the last great contributions of one the most influent theoretical physicists of the 20th century. He had a groundbreaking impact on theoretical atomic and nuclear physics. Quantum mechanics (one of the two pillars on which current theoretical physics is based, along with relativity theory) was strongly related to his research and to the school of theoretical physics he built in Copenhagen. The exact role of the various actors and the influence of the underlying philosophy is still the object of heated controversy in both history and philosophy of science. In any case, Niels Bohr was without a doubt one of the leading actors in the deep transformations from classic to quantum physics, occurring in the first half of the 20th century.

Bibliography

Crockcroft J. D. (1963) Niels Hendrik David Bohr, 1885-1962. Biographical Memoirs of Fellows of the Royal Society 9: 36-53.
Darrigol O. (1992) From c-numbers to q-numbers. University of California Press, Berkely.
Heilbron J. L. and Kuhn, T. S. (1969). The Genesis of the Bohr Atom. Historical Studies in the Physical Sciences 1: 211-290.
Jammer M. (1966) The Conceptual Development of Quantum Mechanics. McGrow-Hill Book, New York.
Pais A. (1991) Niels Bohr’s Times: In Physics, Philosophy, Polity. Clarendon Press, Oxford.
Rosenfeld L. (2008) Bohr Niels Hendrik David, Complete Dictionary of Scientific Biography Vol. 2. Charles Scribner’s Son, Detroit: 239-254