Prof. Dr. Julian Schwinger > Research Profile

by Roberto Lalli
Julian Seymour Schwinger
Nobel Prize in Physics 1965 together with Sin-Itiro Tomonaga, Julian Schwinger, Richard P. Feynman "for their fundamental work in quantum electrodynamics, with deep-ploughing consequences for the physics of elementary particles".
Julian S. Schwinger has been one of the most influential theoretical physicists of the post-World War II era. The techniques he developed have become a substantial part of the common theoretical tools currently employed by physicists, including the quantum action principle, the proper-time method, and the effective action techniques. His best-known work is the renormalisation theory developed between 1947 and 1953, which made it possible to overcome several difficulties encountered by the quantum field theory of electrodynamics in the previous decades. For this work, he was awarded the Nobel Prize in 1965 along with R. Feynman, and S.-I. Tomonaga. While all these theorists plus F. Dyson made fundamental contributions that led to the revival of relativistic quantum electrodynamics, Schwinger was the first to demonstrate that renormalized quantum electrodynamics was in excellent agreement with discoveries recently made, such as the Lamb shift and the anomalous magnetic momentum of the electron. Schwinger also promoted a research program directed towards the unification of electromagnetism and weak interactions. His fecund activity as a researcher was equated only by his teaching ability. His lectures were extremely rigorous and clear, and many eminent scientists, including four Nobel laureates, earned their PhD under his supervision. His approach to problems has been described as conservative and phenomenological. These two features strongly shaped his approach to quantum electrodynamics and to the resolution of its problems. While many physicists believed that it was necessary to formulate a revolutionary theory, he strictly followed the principles of special relativity and quantum mechanics showing that the divergences of the theory could be isolated and eliminated. The same philosophical outlook led later him to criticize the theoretical bases of the Standard Model, especially, the quark hypothesis. This view led him to become an outsider in the last part of his life when he continued to promote an alternative approach to particle physics based on his own source theory.
A Precocious Devotion for Physics
Julian S. Schwinger was born in Manhattan on 12 February 1918, as the second child of a middle class Jewish family. Both his father and his mother's parents had become successful clothing manufacturers after having emigrated from Europe in the last decades of the 19th century. Schwinger began his secondary education in the aftermath of the 1929 Stock Market Clash. The crisis had serious consequences for the businesses of Schwinger’s family, but they managed to overcome the economical difficulties because a different company soon hired Schwinger’s father. At the age of 14, Schwinger enrolled at the Townsend Harris High School, a New York selective public school that usually allowed students to enter the public City College of New York after three years of study. His teachers and schoolmates were impressed by Schwinger’s uncommon skill in mathematics and physics, and many soon referred to him as a genius. After only two years, Schwinger graduated from Townsend Harris and was admitted to the City College. When he was a freshman, he wrote his first paper on theoretical physics at the age of 16. Interestingly, this paper dealt with the very same subject that would gain him the Nobel Prize some 20 years later: quantum electrodynamics. Schwinger elaborated on an equation of Dirac, Fock and Podolsky for describing relativistic quantum electrodynamical interactions between many electrons, which was later called “many-time formalism.” Schwinger generalized the procedure to the case in which the charged particles were quanta of the Dirac relativistic field. The paper – unfortunately, published only posthumously - revealed Schwinger’s early commitment to relativistic quantum electrodynamics and its theoretical setbacks.
The years spent at the City College were very difficult. He concentrated exclusively on mathematics and physics, almost ignoring the other subjects. On the same token, he preferred spending time in the library reading recently published scientific papers than attending classes. Schwinger’s behaviour was seriously affecting his grades and, consequently, his career opportunities.
Being aware of Schwinger’s ability in physics, Isidor Rabi exerted pressure in order to have Schwinger transferred to the Columbia University. Thanks to Rabi’s efforts, Schwinger could officially enrol at Columbia in 1935, where Schwinger could dedicate his entire time to physics and mathematics. The change resulted in a copious production of papers principally dealing with nuclear physics – Rabi’s main area of research. Even before concluding his Bachelor’s degree, Schwinger produced a number of papers on the magnetic scattering of slow neutrons by atoms, which essentially constitute his dissertation. After receiving his B.S. in 1936, Schwinger continued his graduate studies at Columbia under the supervision of Rabi. Apart for a semester spent at the University of Wisconsin to work with the theoretical physicists G. Breit and E. Wigner, he completed his formation at the Columbia University were he earned his PhD in 1939 when he was 21 years old. During this period, he fully developed the peculiar features characterising his research style. He became particularly inclined to work by himself on theoretical problems. This characteristic was strengthened by assuming the habit of working at nights and sleeping during the usual working hours - a routine that allowed him to have just brief meetings with his colleagues. Still more important, Rabi’s dislike for theoretical works without clear contacts with experimental outcomes deeply influenced Schwinger’s approach. As many recognised, Schwinger was always very careful to provide theoretical results comparable with experiments – an approach that would become important also in his work on quantum electrodynamics. This style became the solid basis for a long and fruitful collaboration with Rabi and the group of experimental physicists working at Columbia.
A Tortuous Path Towards Quantum Electrodynamics
After graduating at Columbia, Schwinger spent two years working with Robert J. Oppenheimer at Berkeley. In this period, his main activity was to make complex calculations concerning nuclear physics. The relationship between Oppenheimer and Schwinger was productive but quite complex because Schwinger did not want to be much influenced by Oppenheimer’s view in the resolution of problems. After having obtained a lectureship at the College of Engineering of Purdue University, the dramatic evolution of the World War II required most physicists to concentrate on war-related activities. Contrary to most American theoretical physicists, Schwinger declined the offer to work at the Manhattan Project on the construction of the first atomic bombs, while preferring to study the theoretical problems of microwave guides related to radar developments being pursued at the MIT Radiation Laboratory – commonly called Rad Lab. Many reasons might have been responsible for this choice, including Schwinger’s preferences for working alone, the possibility to collaborate again with Rabi at the Rad Lab, and possible ethical considerations. Whatever the reason, his work on the engineering problems of microwaves turned out to be crucial in his later research on quantum electrodynamics. Historians of science have speculated about at least two strongly interconnected aspects of Schwinger’s work at the Rad Lab that might have led to his following Nobel-awarded enterprise. The first aspect is that the electromagnetic problems related to microwave guides and his successive work on the theory of synchrotron radiation had stimulated Schwinger’s interest in the foundational issues affecting electrodynamics. Since his unpublished first paper written in 1934, Schwinger had not shown any further interest – at least in print – for this topic. Through his war-related researches, Schwinger re-focused on a problem the he had left aside by pursuing other research streams. The second aspect is even deeper. During his theoretical work at Rad Lab, Schwinger actually developed a calculation procedure for electromagnetic phenomena dealing with relativistic velocities. This procedure was essentially based on the employment of the “invariant proper-time formulation of action.” This same approach would be later developed to build a manifestly covariant quantum field theory of electrodynamics and to solve the problems that were affecting such a theory since its foundation in the late 1920s.
Quantum Electrodynamics and the Divergence Problems
Quantum electrodynamics was born at the beginning of 1927, when Dirac published a paper in which he extended non-relativistic quantum mechanics to include the interaction between matter and the electromagnetic field. The aim of the paper was to derive the relationship between the probability of spontaneous emission and the probability of induced emission and absorption. To accomplish this task, Dirac studied the interaction between an atom in an excited state and an external electromagnetic field by applying the perturbation theory to isolated systems. This paper laid the foundation for the developments of quantum electrodynamics. The general approach – the perturbation theory – has been maintained as the main line of attack to quantum electrodynamics problems ever since. In addition, Dirac introduced the concept of “second quantization” of a continuous space-time field. Through the quantization of the electromagnetic field, Dirac derived two operators whose outcomes were respectively the creation and annihilation of a photon. The emission and absorption of photons by the interacting atom was then related to the probability of creation or annihilation of photons. One of the main successes of Dirac’s method was that it led to the correct derivation of Einstein’s formula relating the coefficients of spontaneous emission to those of induced emission and absorption.
In 1927-1928 Jordan and other theorists extended Dirac’s method in order to quantise the matter fields or, more precisely, the space-time wave function appearing in the Schrödinger equation. The outcome of this program was the institution of quantum field theory. Elementary particles, such as the electron and the proton, were not interpreted as ahistorical, permanent, objects, but they might be created and annihilated, being the quanta of underlying fields in space and time. This approach was further developed in 1929-30 by Heisenberg and Pauli and, in a few years, led to the Fermi theory of beta decay based on the creation of electrons as well as to the Yukawa meson theory of strong interactions.
In the meantime, the formulation of relativistic quantum mechanics was pushing many theorists to develop a complementary research program for the description of quantum electrodynamics phenomena. In the first months of 1928, Dirac published his formula for describing spin ½ particles in a relativistic fashion. Dirac’s formula was received with much scepticism. While being able to describe the spin of electrons in a consistent way, Dirac’s formula predicted states with negative energy that could not be ignored. If such states existed, nothing prevented the electron to jump to these energy states. To escape this difficulty, in 1931 Dirac proposed that the vacuum was constituted by negative energy states completely filled by electrons. In virtue of Pauli exclusion principle, one electron with positive energy could not jump in a status with negative energy. The inverse process, however, was not ruled out if enough energy was available. Dirac predicted that when an electron in the negative energy state jumped in a state with positive energy it left a hole in the see of electrons constituting the vacuum. This hole corresponded to a particle with positive charge and could in principle be detected. The “hole” theory of Dirac received a strong confirmation in the period 1932-1933 when Carl Anderson, almost accidentally, observed a particle that seemed to behave like an electron with positive charge.
Anderson did not relate his purely empirical discovery to the theory of Dirac, but others did. Few months later, Blackett and Occhialini corroborated the discovery of the new particle and expressly linked the detected particle to Dirac’s hole theory. In a few months, this discovery and other precise predictions based on Dirac’s theory convinced many physicists of the validity of the Dirac equation. The Nobel Prize awarded to Anderson in 1936 “for his discovery of the positron” sealed this changing perspective of the physics community.
As the historian of science Silvan Schweber put it, since the late 1920s two different research programs began to compete for a reliable description of quantum electrodynamics: the hole-theoretical approach – whose basic ontologies were the particles - and the quantum field theoretical approach – based, instead, on space-time fields. Both of these parallel approaches had serious - and similar - problems. It was understood from the outset that the perturbation worked only if one stopped the calculation to the first order of the coupling constant – namely, the electric charge. If one considered higher orders, overwhelming divergences emerged that made the entire quantum electrodynamics scheme meaningless.
The first divergence to be isolated by studying the second-order term of the perturbation theory was the self-energy of the electron – a divergence that had strong resemblance with the classical problem of the electrostatic energy of a point-charge at rest. Second-order calculations corresponded to a situation in which between the initial and the final states there were intermediate virtual states, which - by virtue of the indeterminacy principle - might have infinite values of energy. In 1930, many physicists - including Oppenheimer, Waller and Heisenberg - emphasised that the perturbation theory necessarily predicted an infinite number of terms due to the interactions of the electron with the virtual photons it emitted. This problem, Oppenheimer pointed out, could not be simply ignored because it had observable consequences: they implied an infinite displacement of the spectral lines of atoms, because the differences between the energy levels were infinite.
After the discovery of the positron, a second type of divergence was recognised: the vacuum polarization. According to the positron theory, the intermediate state included the formation of virtual electron-positron pairs, which might in principle have any value of energy. In 1934, many theorists - including Dirac, Heisenberg, Peierls, as well as Furry and Oppenheimer - pointed out that the vacuum acts as a polarizable medium. An external electromagnetic field induced charge-current fluctuations, which in turn affected the charge originating the electromagnetic field. Also in this case, the integration over all the possible terms of the virtual electron-positron pairs led to infinities. In addition, the application of both the hole theory and the quantum field theory to in principle observable quantities - such as the scattering of electrons by atomic electric fields – showed different kind of infinities. Since 1931, it was calculated that the contribution of radiative effects implied infinite cross section for large wavelengths – an effect called “infrared catastrophe.”
Several ways were attempted to deal with these shortcomings. By manipulating the infinities and the connections between the self-energy of the electron and the self-energy of the photons (vacuum polarization), Weisskopf demonstrated that the self-energy were only logarithmically infinite to any order of the perturbation theory; namely, to any order in an expansion of the self-energy in powers of the fine structure constant alpha=e^2/hc. Moreover, Dirac and others recognized that the effect of the vacuum polarization (including its infinite part due to high energy electron-positron pairs) was to reduce the original charge of a constant factor. In other words, the observable charge of the electron is just the sum of the charge of the electron entering the original Dirac equation plus the effect due to the screening of the polarized vacuum. By discussing this screening effect, in 1936 Robert Serber introduced the term “renormalization” to describe this inclusion of infinite effects into the redefinition of the charge – a term that has been used ever since. Around 1938, analyses of the infrared catastrophe led other physicists, including Pauli, Fierz and, especially, Kramers to propose that the self-energy of the electric field surrounding the charge had the effect to produce an electromagnetic mass. They stressed that both this mass and the original mechanical mass appearing in the Lagrangian were unobservable; only the sum of the original mass and the electromagnetic mass was empirically testable. Between 1938 and 1940, Kramers further developed a non-relativistic theory of quantum electrodynamics in which the essential features of mass renormalization were worked out.
Summarising, by end of the 1930s many ingredients that would appear in the future resolution of the problem were already partially developed. Nevertheless, nobody was able to put forward a relativistic divergent-free formulation of quantum electrodynamics before the works of Tomonaga, Schwinger, Feynman and Dyson in the 1940s. On the contrary, Pauli even snubbed such procedures by defining them “subtraction physics.” The leading theorists of the physics community were sceptical about the soundness of the entire quantum electrodynamics program. The divergences were considered as revealing an internal inconsistency of the theory. As a consequence, the general impression was that relativistic quantum electrodynamics was a provisional theory that worked only in a range of energies inferior to 137mc^2; namely, when the wavelength of the wave function of the electrons is of the order of the classical electronic radius, and many thought that a new revolutionary theory was necessary to deal with higher energies.
A Conservative Approach to Quantum Electrodynamics: The Triumph of Renormalization Theory
World War II completely transformed the world of physics in many different ways. In the United States, the fundamental role of physicists in the construction of the atomic bomb made this transformation even more radical. After the war, the community of American physicists enjoyed strong economic support as well as novel techniques and facilities for pursuing their researches. Moreover, the formation of interdisciplinary teams led to stronger collaborations between theorists and experimentalists. One of the main outcomes of the war efforts was the possibility to use completely new devices developed for war-related activities. In addition, physicists working in military laboratories have developed a strong expertise in the employment of such instruments. In the United States, this knowledge played a crucial role in the revival of quantum electrodynamics in the 1940s.
Two important experiments were realized with techniques produced in connection with radar development researches. The first of these experiments was concluded already in April 1947. Willis Lamb, along with his graduate student Robert Retherford, found that there was a energy difference between the first two excited states of the hydrogen atom - the levels 2S1/2 and 2P1/2. The frequency of 2S1/2 turned out to be higher than that of the 2P1/2 by about 1000 Mhz - a result that was soon christened Lamb shift. This separation was in contradiction with the relativistic Dirac theory of the electron, according to which the two levels should be equal. Some physicists had reported similar discrepancies previously, but Lamb’s methodology was considered so reliable that his experimental result turned out to be a fundamental push for reconsidering the theory of quantum electrodynamics.
To maintain connections between theorists after World War II, the National Academy of Sciences promoted a series of conferences to discuss fundamental issues in theoretical physics. The first of such conferences was held at the Shelter Island from 2 to 4 June 1947 and saw the participation of 23 theoretical physicists, including Schwinger. During the conference, Lamb reported his result and various hypotheses were advanced as for the reason underlying the 2S1/2-2P1/2 shift. Schwinger, along with Weisskopf and Oppenheimer, suggested that the Lamb shift might be the finite effect of the interaction of the electron with the radiation field.
Following these suggestions, Hans Bethe made soon a calculation in a non-relativistic framework. Bethe’s reasoning was based on the renormalization of the electronic mass: the divergent self-energy to second-order of the perturbation theory was integrated in the redefinition of the observed mass. The observed mass m was considered as the sum of the bare mass plus the electromagnetic mass. The reasoning was based on the idea that the only meaningful mass term was the observable mass and, consequently, that the bare mass should be eliminated by all the equations. Bethe found the final value of shift due to the interaction by subtracting the self-energy of the free-electron to the self-energy of the bound electron. Bethe’s calculation led to a result that agreed pretty well with the observed Lamb shift. This gave some physicists the hope that divergences in quantum electrodynamics could be eliminated and that the radiative effects might be calculated. Bethe’s result, however, was based on a non-relativistic approximation and on the assumption that a relativistic calculation based on the Dirac theory would lead to a natural cut off at energies K=mc^2.
At the Shelter Island Conference, another experimental anomaly was reported. Rabi and his students J. E. Nafe and E. B. Nelson had observed a serious discrepancy in the hyperfine structure of hydrogen and deuterium. The empirical result did not fit with the predictions of the Dirac’s theory, and G. Breit soon proposed that it might be related to an anomalous magnetic moment of the electron. In the following weeks, P. Kusch and H. M. Foley confirmed Breit’s idea of the anomaly in the intrinsic magnetic moment of the electron by observing the splitting of the hyperfine structure of heavier atoms. The experimental discoveries prompted new interest towards the finite effects of quantum electrodynamics and their importance was recognised with the 1955 Nobel Prize awarded to W. Lamb “for his discoveries concerning the fine structure of the hydrogen spectrum” and to Kusch “for his precision determination of the magnetic moment of the electron.”
After Bethe had made his first non-relativistic calculation, Schwinger began looking closely at the problem guided by the recent empirical discoveries. He first developed a model using successive canonical transformations to derive the anomalous magnetic moment of the electron through renormalization of charge and mass. Albeit correct in giving the result actually reported by experimenters, the model was non-covariant and was soon substituted by Schwinger’s second theory of quantum electrodynamics. In a handful of months, he devised a fully covariant and gauge invariant formulation of quantum electrodynamics that isolated the divergent terms and cancelled out them by means of the renormalization of mass and charge. The techniques Schwinger employed were still based on a series of canonical transformations, following the paper of Dirac, Fock and Podolski he had already worked on when he was 16 years old. By means of such canonical transformations, Schwinger eliminated the virtual effects. In this version, Schwinger presented a manifestly Lorentz covariant generalization of the Schrödinger equation to an arbitrary space-like surface:
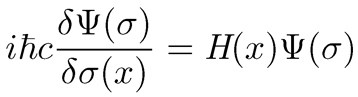
where H is the interaction energy density at the point x of space-time x, and σ(x) is a general 3-dimensional surface in space-time. This equation came to be known as the Tomonaga-Schwinger equation because Tomonaga had first derived it in 1943. Although the Japanese theoretical physicist had found it during the war, Schwinger was actually the first to successfully employ renormalization procedures to obtain finite results, such as the Lamb effect and the anomalous momentum of the electron.
Schwinger presented his manifestly covariant theory at the second conference on the fundamental problems of theoretical physics held at the Pocono Manor Inn in the Pocono Mountains in Pennsylvania in 1948. The first day of the conference was almost a one-man show in which Schwinger made all the complex and long calculations for several hours. The covariant formulation given by Schwinger led to the development of a consistent quantum electrodynamics, isolating and removing the self-energy of the electron by mass renormalization and the divergences linked to the vacuum polarization by means of charge renormalization. After eliminating all the divergences at the first order of alpha, Schwinger derived the Lamb shift. The attendees of the Pocono conference were enthusiastic about Schwinger’s approach, and relativistic quantum electrodynamics, along with the complex renormalization procedures, became a main research field in the following years. At the same conference, Feynman also presented his method based on the Feynman diagrams and the path integrals, but the reception was quite negative. It was Freeman Dyson who in 1949, even before Feynman published his own papers, demonstrated that the two approaches were equivalent. In another paper, Dyson also showed that in both Schwinger’s and Feynman’s theories the divergences were cancelled out for any order of the constant alpha.
In the months following the Pocono conference, Schwinger published a series of three foundational papers called “Quantum Electrodynamics I, II, and III,” in which the entire theory and its consequences were thoroughly discussed. The renormalization procedures - above all in the simplified form represented by the Feynman diagrams and rules - spread out and became useful tools for calculating the corrections due to higher-order virtual states. At the same time, it was possible to regard renormalization as an essential prerequisite for a quantum field theory to be acceptable. Thus, renormalization became a criterion underlying the choice between different theories and different research programs.
Although unconvinced by Feynman’s approach, Schwinger recognized the usefulness of employing different procedures in order to deal with higher-order terms. Since 1949, Schwinger began exploring an alternative version of quantum electrodynamics based on the quantum action principle – a generalization of the least action principle in classical mechanics – which, according to some, provided the most rigorous approach to quantum field theory. In spite of the importance of the formulation of renormalized quantum electrodynamics and the formidable agreement with empirical observations, the Nobel Prize was conferred only many years later. In 1965, the Swedish Academy awarded Schwinger, along with Feynman and Tomonaga, the Nobel Prize in Physics “for their fundamental work in quantum electrodynamics, with deep-ploughing consequences for the physics of elementary particles.” After the Nobel Prize was already awarded, other events further amplified the relevance of their work. While until 1971 most physicists considered the relativistic quantum field theory as the right framework for quantum electrodynamics alone, from the early 1970s the renormalized quantum field theories of electroweak and strong interactions received strong empirical confirmations and became the Standard Model of particle physics.
Schwinger’s Precursory Work on Electroweak Synthesis
While working at the renormalization of the quantum field theory of electrodynamics, Schwinger developed techniques that became standard theoretical tools broadly employed by theorists. His influence on the community of theoretical physicists was also due to his productive activity as a teacher and PhD supervisor. His Harvard lectures became legendary for clarity of exposition. In addition, he trained several physicists and supervised more than seventy dissertations. Some of his students made fundamental researches, often related to the work began under Schwinger’s direction, as in the case of the development of the electroweak theory.
In 1957, Schwinger was the first physicist to propose the unification of the electromagnetic and weak interactions under the same mathematical structure: the SU(2) gauge symmetry group. This group implied the existence of three different force carriers, the massless photon being the quantum of the electromagnetic field. The others were two massive charged particles that were supposed to mediate the weak interactions. His student S. Glashow inherited Schwinger’s belief that there should be a unified description of weak interactions and electromagnetism, and further developed Schwinger’s original proposal. In 1961, Glashow overcame some of the difficulties of Schwinger’s theory by suggesting that the symmetry gauge was, instead, SU(2)xU(1), which predicted the existence of four force carriers. Weinberg and Salam later developed this proposal by means of the Higgs mechanism for explaining the masses of the weak force mediating bosons. After the model was proved to be renormalizable by ‘tHooft and with strong empirical confirmations, the Glashow-Weinberg-Salam theory became accepted as the Standard Model of electroweak theory during the 1970s.
Towards the Fringe
In spite of the importance of Schwinger’s work in providing the theoretical tools physicists still learn in graduate classes and in spite of training several first rate physicists, Schwinger began to be considered an outsider since the early 1970s. He had always tried to reformulate quantum electrodynamics because he had never been convinced of the completeness of the theory. During the 1950s and the 1960s, Schwinger tried to develop the quantum field theory in order to include weak and strong interactions. Soon after having received the Nobel Prize, the difficulties of integrating the strong interactions in quantum field theory led Schwinger to propose a completely different approach, called source theory - a theoretical perspective based only on observables. While before, Schwinger and his students were among the principal proponents of the extension of quantum field theory to include all the particle interactions, in 1965 his phenomenological stance led him to dislike theories based on unobservable entities, such as matter fields and quarks. When quantum field theory became the mainstream approach for the description of particle interactions and quantum chromodynamics became accepted by most physicists in the early 1970s, Schwinger did not change his philosophical perspective and continued to develop his source theory with the collaboration of his students.
This situation created a split between Schwinger and the mainstream community of theoretical physicists - a separation that became even stronger when Schwinger showed much interest for the alleged discovery of cold fusion and for the quantum field theoretical explanation of sonoluminescence. In 1971, Schwinger also left Harvard University for the University of California at Los Angeles, where he died in 1994 after some public controversies with physicists and journal editors had further distanced him from his colleagues. The discordance between Schwinger’s relevance and the last part of his career – when his views were considered by most as outdated – was not uncommon among practitioners of physics, and resembled closely that of Einstein who continued to criticize the foundations of quantum mechanics and, in particular, of the Copenhagen interpretation well after the theory had been accepted by the majority of physicists. Both Einstein and Schwinger concluded their career at the fringe of the field they had contributed to build in a fundamental way.
Bibliography
Brown, L. M., Dresden, M., & Hoddeson, L. (Eds.) (2009) Pions to Quarks: Particle Physics in the 1950s. Cambridge University Press, Cambridge.
Fitch V. L., & Rosner L. (1995) Elementary Particle Physics in the Second Half of the Twentieth Century. In Brown, L., Pippard, B., & Pais, A. (Eds.). Twentieth century physics (Vol. 2). AIP, New York, pp. 635-794.
Mehra, J., & Milton, K. A. (2003) Climbing the mountain: the scientific biography of Julian Schwinger. Oxford University Press, Oxford.
Pais, A. (1986) Inward Bound Of Matter And Forces In The Physical World. Clarendon Press, Oxford.
Schweber, S. S. (1994) QED and the men who made it: Dyson, Feynman, Schwinger, and Tomonaga. Princeton University Press, Princeton.
Schwinger, J. (Ed.) (1958) Selected papers on quantum electrodynamics. Courier Dover Publications, New York.
